八階三角形鑲嵌
外观
 龐加萊圓盤模型 | ||
| 類別 | 雙曲正鑲嵌 | |
|---|---|---|
| 對偶多面體 | 正八邊形鑲嵌 | |
| 識別 | ||
| 鮑爾斯縮寫 | otrat | |
| 數學表示法 | ||
| 考克斯特符號 | ||
| 施萊夫利符號 | {3,8} | |
| 威佐夫符號 | 8 | 3 2 4 | 3 3 | |
| 組成與佈局 | ||
| 頂點圖 | 38 | |
| 對稱性 | ||
| 對稱群 | [8,3], (*832) [(4,3,3)], (*433) [(4,4,4)], (*444) | |
| 圖像 | ||
| ||
在幾何學中, 八階三角形鑲嵌 是由三角形組成的雙曲面正鑲嵌圖,每八個三角形共用一個頂點。在施萊夫利符號用{3,8}表示。八階三角形鑲嵌即每個頂點皆為八個三角形的公共頂點,頂點周圍包含了八個不重疊的三角形,一個三角形內角60度,八個三角形超過了360度,因此無法因此無法在平面作出,但可以在雙曲面上作出。
正塗色
[编辑]如要得到一半的對稱性[1+,8,3] = [(4,3,3)]可透過將三角形以兩種顏色交錯塗色而得到。
對稱性
[编辑]



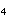 .
.在[(4,4,4)] 對稱性中, 有十五個(七個特殊)透過移除鏡射線和交錯變換的子群。 若一個鑲嵌中的階數為偶數,則可移除鏡射線,並將相鄰、分開的階去除一半。移除兩條鏡射線會變成半階偏轉點,其為移除鏡射線交會的地方。 在這些圖像中,基本域交替著色為黑色和白色, 而鏡射線位於兩種顏色交會的邊上。 在每個基本域上添加三個平分鏡射線會產生832對稱性。 子群指數-8群, [(1+,4,1+,4,1+,4)] (222222)是[(4,4,4)]的換位子群。
一個更大的子群構造為[(4,4,4*)],指數為8,可作為將部分頂點去除的(2*2222),也就是(*22222222)。 此對稱性可透過加入平分基本域的鏡射線增倍為842對稱性。 此對稱線可由6擴展,透過在每個域加入三個平分鏡射線,作為832對稱性。
| 子群指數 | 1 | 2 | 4 | |||
|---|---|---|---|---|---|---|
| 圖形 | 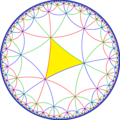
|

|
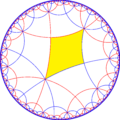
|
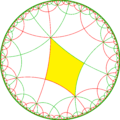
|

|

|
| 考斯特圖 | [(4,4,4)] |
[(1+,4,4,4)] |
[(4,1+,4,4)] |
[(4,4,1+,4)] |
[(1+,4,1+,4,4)] |
[(4+,4+,4)] |
| 軌形 | *444 | *4242 | 2*222 | 222× | ||
| 圖像 | 
|

|

|
|

| |
| 考斯特 | [(4,4+,4)] |
[(4,4,4+)] |
[(4+,4,4)] |
[(4,1+,4,1+,4)] |
[(1+,4,4,1+,4)] | |
| 軌形 | 4*22 | 2*222 | ||||
| 導向子群 | ||||||
| 指數 | 2 | 4 | 8 | |||
| 圖像 | 
|

|

|

|
| |
| 考斯特 | [(4,4,4)]+ |
[(4,4+,4)]+ |
[(4,4,4+)]+ |
[(4+,4,4)]+ |
[(4,1+,4,1+,4)]+ | |
| 軌形 | 4242 | 222222 | ||||
| 根源子群 | ||||||
| 指數 | 8 | 16 | ||||
| 圖像 | 
|

|

|

|

|

|
| 考斯特 | [(4,4*,4)] | [(4,4,4*)] | [(4*,4,4)] | [(4,4*,4)]+ | [(4,4,4*)]+ | [(4*,4,4)]+ |
| 軌形 | *22222222 | 22222222 | ||||
相關多面體及鑲嵌
[编辑]
| 多面体 | 欧式镶嵌 | 双曲镶嵌 | |||||||
|---|---|---|---|---|---|---|---|---|---|
 {3,2} |
 {3,3} |
 {3,4} |
 {3,5} |
 {3,6} |
 {3,7} |
 {3,8} |
 {3,9} |
... |  {3,∞) |
| 球面 | 雙曲鑲嵌 | |||||||
|---|---|---|---|---|---|---|---|---|
 {2,8} |
 {3,8} |
 {4,8} |
 {5,8} |
 {6,8} |
 {7,8} |
 {8,8} |
... |  {∞,8} |
在Wythoff構建中,有十個雙曲正鑲嵌可以由正八邊形鑲嵌以及八階正三角形鑲嵌構造而來。
此外,八階三角形鑲嵌作為一種無窮抽象多胞形,可以具象化為一種扭歪無限面體,該扭歪多面體皆由三角形組成,每個頂點都是8個三角形的公共頂點,其結構可以看做是依照立方晶格排列的扭稜立方體移除其正方形面所構成的幾何結構。[1]
參見
[编辑]參考資料
[编辑]- ^ Richard Klitzing. hyperbolic order 8 triangular tiling : otrat. 3D convex uniform polyhedra. bendwavy. [2021-09-05]. (原始内容存档于2022-12-10).
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- Chapter 10: Regular honeycombs in hyperbolic space. The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.




